Here, at least, is one case where I have a complete set of stellations – those of the Truncated Octahedron. Complete, that is, in the sense of closed, connected stellations. Like Coxeter and Wenniger writing about the icosahedron, I can see other stellations with holes through the middle, and disconnected pieces; and there’s also an infinite one – but we’ll come back to them later. Here, because they make a nice intellectual journey, are the Truncated Octahedron and the four basic stellations we obtain by extending its sides:
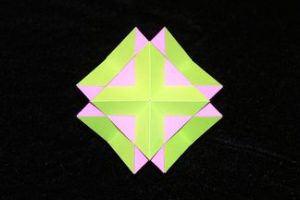
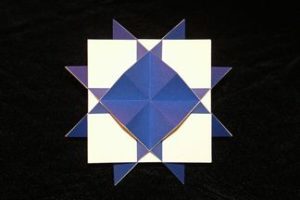
First Stellation of the Truncated Cube
To stellate the basic shape we extend the hexagons out over the squares (which simply restores the original points of the original octahedron), and we extend the squares out over the hexagons (which produces something a bit like a cube structure, but rather more complex. I chose not to use the same colours, so there’s a little extra work involved in understanding it all here; but it should be clear enough…
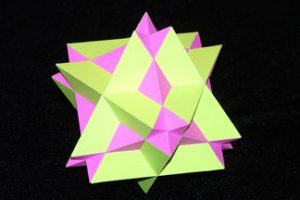
The Second Stellation of the Truncated Octahedron
The first stellation is a beautiful shape in its own right, but even better is to come when we extend the faces again. It’s easy to see the blue pairs of triangles will get filled in with the red sides extending across them, but it’s harder to work out what happens with those red pointy corners and the blue pyramids. The spaces between them get filled up, but there are also cute little extensions over the red ends…
Those purple corner pieces took me ages to work out geometrically, and were a nightmare to produce in reality– there are six of them in the model, but I must have thrown away about another six of them while I was making it. But look carefully and you can convince yourself – they are indeed the right extensions of those red faces in the first stellation.
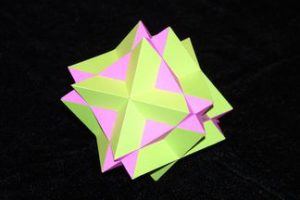
Third Stellation of the Truncated Octahedron
I did a little dance of joy when I completed the second stellation, but that was nothing compared with the awesome beauty of the third. Perhaps I just got the colours particularly right, but what a cracker this one is! It has lovely big triangular parts (blue) which sweep through, combined with six stunning white squares. And as always there are at least 3 completely different “orthogonal” views – centred on the triangular face, the square face, and an edge view.
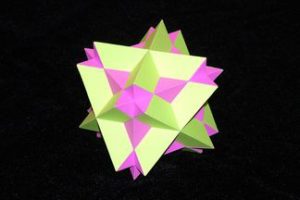
Fourth Stellation of the Truncated Octahedron
The last of these four stellations is a strange beast, and I have to say I wasn’t quite convinced it was even a valid one. But I checked with Wenniger, found he faced exactly the same problem, and ploughed on regardless. And it certainly meets Coxeter’s criteria as discussed below – so here we go.
The “problem” relates to the way we’ve been discovering each of these shapes – essentially we’ve done it extending the faces of the existing shape and working out where they meet. I think of this as ‘stellation’, but a better word might be ‘extension’, meaning a subset of all the possible stellations.
Since the original truncated octahedron has a total of 14 faces, this suggests each face will meet 12 others (leaving out, of course, itself and the one directly opposite.) This means the square faces will meet the other 12 in 3 sets of 4, while the triangular (hexagonal) faces will meet the other 12 in 4 sets of 3 – and herein lies the problem. Once we’ve worked through 3 of these extensions there are no more to come for the square faces, yet the hexagonal faces still have a step to go; so our 4th stellation looks just a bit like the 3rd. In particular, those big white square patches are still visible as parts of the green chevrons: they haven’t yet disappeared into the interior of the solid, though other parts have been extended – notably the little yellow points and the big green/yellow peaks.
And is it quite as beautiful as the others? In some ways I think it’s less complex, and duller – though it was trouble enough to build. But it’s still attractive – and, overall, what a fabulous collection! I’m thrilled to bits to have discovered, and to have made, these.
The only trouble is…we’re not done yet…
The other Thirty or so Stellations of the Truncated Octahedron
And now for the bad news. We’ve drawn up the familiar line diagrams showing where all the faces intersect, and we note that these diagrams are full of polygons: in 3 dimensions these polygons define lots of cells which combine to form the stellated solids. We’ve worked our way through them by extending outwards at each stage – essentially grabbing as much “new turf” as we could deal with. And we’ve reached the absolute outer limit of where these stellations can take us: there can be no other solids in this collection which are bigger than the green and yellow one above.
But mathematically, the definition of ‘stellation’ is broader than this: it allows for any combination of the internal cells, with only the most obvious restrictions: like the cells must all be the same, they should join together coherently, etc etc. This leads us to a much bigger set of stellations – including some with holes through the middle, and many which merge different features of the existing ones. There may even be some “snub” (i.e. right- or left- handed) ones, though I haven’t identified any “snubs” yet. So far (and I’m not convinced I’m finished) I reckon I can name at least 30 of these…yes, 30!
I see another workplan for the next 10 years…and a need to buy more cupboards!
And more awaits us. There would be plenty of fun in staying with the archimedean solids and their stellations, but even better is to come when we look at their Duals – the Catalan Solids. We’ll start gently with a manageable look at Stellations of the Rhombic Dodecahedron.