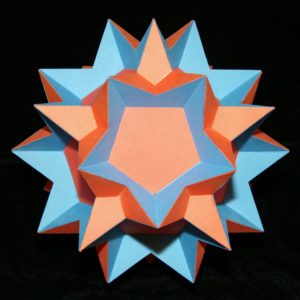
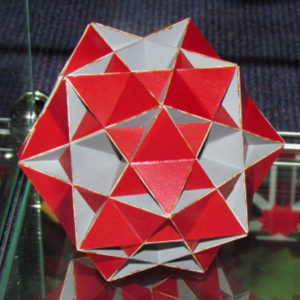
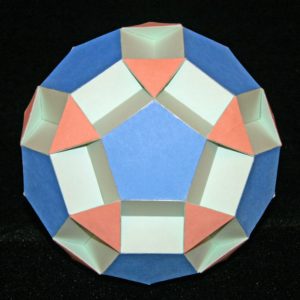
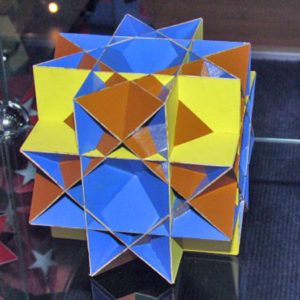
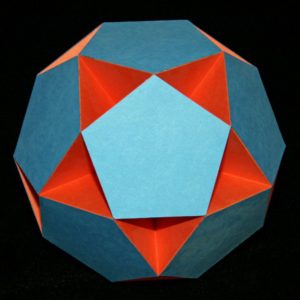
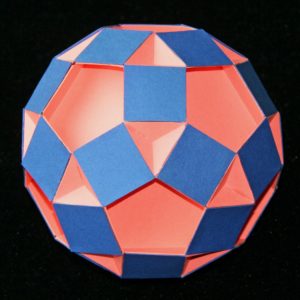
These at last are the most wonderful polyhedra of the lot. There are 53 to consider here, which is such a large number that I don’t think I’ll ever run out of love for them: they’re just staggering, mind-blowing, amazing. I could spend the rest of my life admiring these shapes.
The basic ideas are all familiar, though we haven’t put them all together yet. From the Platonics – every vertex should be the same; from the Archimedeans – there can, however, be a combination of different polygons round each vertex; then from the Kepler-Poinsots the two ideas of letting the polygons penetrate through each other, and also of star polygons.
The polygons we’ll work with here are our old friends the triangle, square, pentagon, hexagon, octagon and decagon; and also the 5-pointed star (pentagram). But for the first time we find we can also use the 8-pointed star (octagram) and the 10-pointed star (decagram).
Here we also start using the numbering W67 etc, which relates to the classic text – Marcus Wenninger’s book Polyhedron Models.
With 53 shapes it’s quite hard to find a way in to understanding them: the gallery above shows them all in Wenninger’s order but they’re listed more systematically below. That ‘system’ is simply my view on the way some of them just look obviously like each other: for instance 68 and 78. So I’ve adopted 3 main criteria: do they fit into the same ‘external shell’; do they follow on from each other by some obvious process like stellation or ‘hollowing-out’; or is there some other idea which connects them.
You can also work through them slowly one by one if you’d like to by following the Details link in the menus. (Not quite there yet)
At the start is one which stands on its own. Tetrahedral symmetry is very simple and produces only the Tetrahemihexahedron here, which obviously sits inside an Octahedron.
The next two look like inverses of each other, and both fit inside a Cuboctahedron. Aside from the obvious squares and triangles on the surface, each has hexagons which slice through the centre of the shape:
Since anything we can do with the Cuboctahedron generally means we can do the same, and better, with the Icosidodecahedron, here’s a lovely pair, both with decagons which slice right through the centre of the shape:
The same idea is here in these two shapes based on the Small Rhombic Cuboctahedron. Squares and Triangles on the surface, and Octagons which cut through the main part of the shape – though not actually through its centre.
…and again we can do this with a Small Rhombic Icosidodecahedron:
At this point we run out of options for simply taking slices through the Archimedean solids: the reason the obvious one that we’ve used up all the Archimedeans where you can clearly do this ‘slicing’. (We’ll see more of this with the 92 Johnson Solids later on. But new shapes come in as prospective ‘external shells’, and ‘hollowing-out’ produces some new options. The first family here comes from an external shell which has 3 stars meeting at each vertex. If you wanted to, you could say the external shell is a dodecahedron, though that observation feels less important than before in defining the new shapes:
Here’s a similar progression, but we start with the stars exploded away from each other and separated by triangles:
And here is the same trick, but we start with a form where the stars are rotated: so instead of 3 points meeting at each vertex, we have 3 stars whose arms meet to surround ‘the space where the vertex used to be’.
As before we can explode the stars away from each other, but this time they separate with squares filling the gaps rather than triangles:
Now we start some work with the ‘big stars’ – the Octagram and Decagram. We start with a shape derived from a cube, and do the usual hollowing-out:
Then, as before, we pull the stars apart – first separating them with squares and then with hexagons; then finally we push them rather violently in:
And as usual, what we can do to the cuboctahedral form we can also do to the icosidodecahedral:
And now there are three simpler forms which are just very obviously Truncated, or Quasitruncated, forms of the Kepler-Poinsot solids.
Then there are a further three which share the external form of the Great Icosidodecahedron, a nice mix of triangles and pentagrams. The two hollowed-out versions show more of the underlying structure, which is basically decagrams slicing through the middle.
A different arrangement of the decagrams brings them more to the surface and shrinks the exposure of the triangles and pentagrams – and by the time we get to hollowing it out there seems very little left…
Before we get to the 10 ‘snubs’ – which are really in a world of their own – there’s one left which seems to stand on its own. You could relate it to the decagrammic ones a few rows up and regard it as a slight ‘implosion’ of one of them, but really it deserves to be treated as a one-off:
Lastly there are no less than 10 snubs and also John Skilling’s Polyhedron (S1), which we’ll include here since it turns out to be a very close relative. Among these there’s only really one family, made up of two of the snubs and Skilling’s Polyhedron. They have very similar external forms, all of them with the distinctive feature of paired pentagrams in the same planes:
John Skilling’s polyhedron is particularly weird in that some of its edges have 4 rather than 2 faces meeting at them. You might think this means some faces are completely inside, which would break the rules; but in fact every face which is internal on one edge pops out and is external on the next edge, so it turns out the shape as a whole is valid.
After these, the snubs are so weird that they don’t really seem to belong in families. I’ll make some comment on them below, but on the whole this feels more like a world of geeky teenagers where ‘families’ don’t really operate.
W110 is always appealing – not only because it’s my own model but because it has a basic simplicity of form – but I don’t think the external form is shared with any other. As for W114, well, it’s just astonishing that it works:
The next two look as if they might have the same vertices – the points of the stars – but there are elements of scaling which make that untrue. Indeed to calculate the vertices of W112 we have to work with the ‘plastic constant’ (1.3247180…) which I must admit I’d never heard of until I came to write this up…
The next three do have the same vertices, but other than that it’s hard to spot any relationship between them. Perhaps I’ll discover one if I dream about them long enough:
117 and 118 both have sharp edges, but apart from that there’s little to connect them: so 118 gets the final place on its own, at the very end of the list:
Can there be any more left after this? Well, yes; and the next set is rather large – it’s The infinite family of Prisms and Antiprisms.