Two important new ideas come in here: one is to use stars as well as the ordinary convex polygons, and the other is to let the polygons lie inside the overall model, or cut across it.
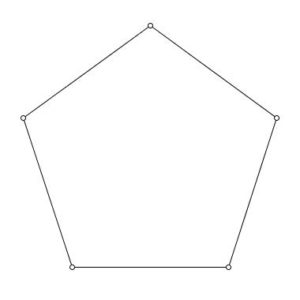
Star Polygons
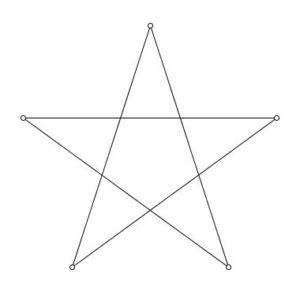
How many pen-strokes does it take to draw a 5-pointed star? Some people would draw round the outside and use 10, but actually you only need 5 if you let your pen run across the star. We call such a star a pentagram, but you can consider it as just a pentagon with its sides crossed over. It’s non-convex, which is a change from what we’re used to, but if it’s still regular (all the sides and angles are equal) then we can use it to build some beautiful new polyhedra.
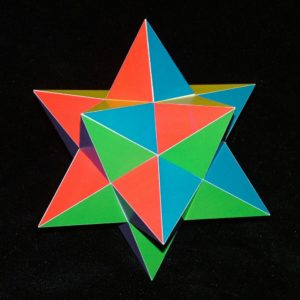
Putting 12 Pentagrams together and letting them cross over at the long ‘edges’ we get an astonishing new shape – the Small Stellated Dodecahedron:
The first impression here is just of a pretty star with 5-sided pyramids as points; but the underlying structure is really formed by the coloured stars. Recall that an edge here runs across the whole model, and it’s quite easy to see the blue and green pentagrams meeting along a whole long edge: indeed the faces don’t just meet, they slice through each other. Then they meet up with the red star in the same way. Eventually, twelve stars make up the whole polyhedron.
Another way to look at this one is to ask what shape is at the centre of it – it’s quite easy to visualise a Dodecahedron and to imagine its faces all extended out to the points where they meet. This process is Stellation: there’s a lot more to say about that later, but this will do for the moment.)
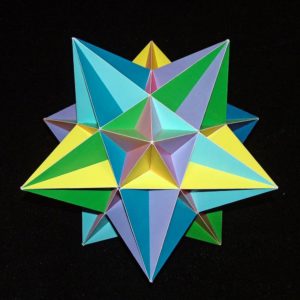
The other polyhedron to consider here is similar but intriguingly different. It also uses 5-pointed stars, but it connects them together in sets of 3 round each vertex instead of 5. Again the stars join along the big sides which traverse the model, but it also looks as if it’s made up of 3-sided pyramids. At its heart is actually an Icosahedron, but because it uses 12 stars it’s again called a Dodecahedron: this time the Great Stellated Dodecahedron.
Non-Convex solids (Self-Intersecting, Cutting Across, Re-entrant)
Johannes Kepler knew about the Great and Small Stellated Dodecahedra back in 1619, but he missed a couple more – they had to wait for Louis Poinsot in 1809. Poinsot made a leap of thought which almost goes backwards: he figured that if we permit pentagrams to intersect each other, then why not also allow our old friends the triangle and pentagon to do the same? These are not star polygons, but perhaps we could let them intersect with each other… and this led him to the Great Dodecahedron and the Great Icosahedron.
Although its triangular dimples might lead you to mistake it for an Icosahedron, the name Dodecahedron is used here because the Great Dodecahedron is made from 12 pentagons. You can see them clearly here in different colours. The important point is that there are no stars here – it’s all just plain old pentagons, despite what looks like a star in the second photograph.
Having found the Great Dodecahedron the search was clearly on to see if there was a Great Icosahedron. As the name suggests, this has 20 triangles and connects them by letting them all interpenetrate. In concept it’s not difficult, but making it from real paper and glue is quite difficult: its very sharp angles and the tiny pieces make it a tough job. I made one in about 1978 with paper and sellotape, but it fell apart sometime along the way; happily these days we can use computers and colour printers, so it becomes a more manageable proposition. Here is the wonderful Great Icosahedron:
Are there any other shapes like these? You might think of investigating squares and even more complex polygons like octagons and decagons. It turns out there are no more regular polyhedra like this, but like we did with the Archimedeans, we can relax the rules to allow more than one kind of polygon at each vertex. This leads to The 53 Semi-Regular Non-Convex Polyhedra.