It’s rather alarming to think there are 53 new Dual models to make here. We might also be put off by the incredible complexity we can expect of some of them, and possibly by the feeling that the Duals are never quite as exciting to look at as the ‘original’ polyhedra.
Happily in some cases the vertices of the Dual lie completely inside and are obscured by other parts of the structure: and since these Duals tend to occurs in pairs or even triads, we can get away with making less than 53 – a quick look suggests we’ll need about 40. We can further divide the work up by observing there are some Dual models that are easy, others that that will be challenging, and some that we’ll never get round to at all – so it’s not worth feeling guilty about them. And given that colour is not such an issue with Duals, perhaps the work reduces to about 10 years.
As an example here is the Small Hexacronic Icositetrahedron (D69), the dual of both the the Small Cubicuboctahedron (W69) and the Small Rhombihexahedron (W86). The photographs below show how similar the main Polyhedra are: it still seems surprising that vertex patterns 8-8-4 and 8-8-3 should produce the same dual, but the reasons lie deep in the ‘intersections’ that are completely internal to the model.
I’ve hardly made many of the others, so we’ll focus mainly here on the subset of Duals of the 9 Hemi-Polyhedra. A Hemi-Polyhedron, as suggested by its name, has faces slicing right through its centre: so this raises an interesting question: given that the Dual process is mathematically about taking the reciprocals of distances to the centre, how can the corresonding vertices appear in the dual when the calculation seems to involve 1/r with r=0?
The answer once you ‘get it’ is obvious: these vertices actually shoot off to infinity, and the mathematics all falls out fairly well. But though the maths is tidy, it’s less easy to build a shape that is infinite!
We handle this with a compromise, again obvious once you ‘get it’ but nevertheless a neat piece of thinking when Wenninger first made it. Since the infinite vertices force the shape to have essentially parallel legs, we simply chop these off at a suitable distance from the centre and then imagine they carry on for ever. I’m skating past lots of mathematical subtleties here, but a picture will help explain what’s going on:
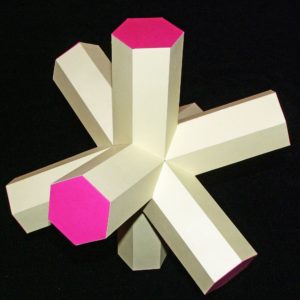
The yellow squares have no function at all except to ‘cap’ the square prisms in our real finite space, though they do helpfully remind us that the ‘legs’ are square in cross-section. But the main point is that this Dual is derived from a Hemi-polyhedron which originally had faces which passed through its very centre. Since there are three prisms there must have been three faces – and since the prisms meet in threes, there must be some other three-ishness about the original. What was that original Hemi-polyhedron? It was the Tetrahemihexahedron, the very first one we encountered among the the Non-Convex Semi-Regular polyhedra.
The important thing here is the green ‘legs’, which you have to think of as shooting off into the far distance to infinity – they never get any wider or thinner, but just travel out endlessly to the furthest parts of outer space. In fact it helps if you can imagine think of them as only 3 legs, not 6 – the idea being that each leg shoots off to infinity and then comes back from the opposite direction. Mathematically there are really only three infinite square prisms intersecting here.
For such a small shape this one is surprisingly tightly connected and really quite weird: it has a strange total of 7 faces, and as we’ve said, the green, yellow and red faces all pass through the centre. In the dual these 3 hemi faces become the 3 vertices at infinity representated by the 3 prisms. As for the blue triangles… well each of them becomes a 3-sided vertex in the central crossing of the Tetrahemihexacron at the conjunction of three light green faces. This seems a rather feeble end for the blue triangles, but there we go.
The second in our set of Duals of Hemi Polyhedra is the first one to be the dual of two originals: the Octahemioctahedron and the Cubohemioctahedron. Because of this it gets two names: the Octahemioctacron and the Hexahemioctacron. It’s basically just 4 hexagonal prisms intersecting each other; but it has a certain pleasantly firm look about it:
If you’ve explored elsewhere on Colinsmaths you might recognise the Octa-Hexa Hemioctacron from our discussion of a Hexastix: you can make it with 72 pencils and 8 elastic bands and it has a charm all of its own.
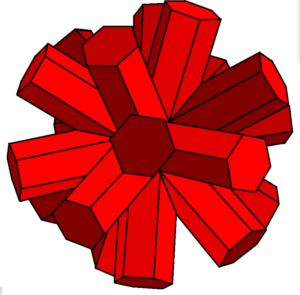
A more advanced example is the polyhedron below, the Small Icosihemidodecacron / Dodecahemidodecacron:
Here the important thing is again the pink legs, which again you have to think of as shooting off into the far distance to infinity – they never get any wider or thinner, but just travel out endlessly to the furthest parts of outer space. The green decagons are merely where we’ve chopped these legs off, and they have no mathematical significance other than to remind us that the legs are decagonal in cross-section. There happen to be 12 of them, and the whole thing obviously has the usual icosahedral/dodecahedral symmetry – though again we have to think of them in pairs: so this is really just a conjunction of 6 decagonal prisms derived from a Hemi-polyhedron which originally had some faces passing through its very centre.
And which was that original Hemi-polyhedron? It turns out there are two, both leading to this same Dual: they’re our old friends the Small Dodecahemidodecahedron and the Small Icosihemidodecahedron.
We can see that both of these have 6 hemi planes with 10 sides (those big orange and/or blue decagons) so these ‘obviously’ become 10-fold vertices at infinity in the Dual. There are 6 of them in each case, and if you make the same jump of considering the ‘legs’ in pairs (so 12 becomes 6) we can see how it all works.
Again, it’s less clear where the blue pentagons and the red triangles go to in the Dual. They ought, in turn, to go to 5-fold and 3-fold vertices somewhere, but in fact they disappear somewhere into the inside and become part of the complex internal structure once the pink legs start intersecting each other. In these days of software rendering there ought to be ways to show this, but I’m not aware anyone has bothered: so we’re rather stuck with Wenninger’s view copied into Mathworld and Wikipedia: that the lovely 10-legged Dual we have here should be called both the Icosihemidodecacron and the Dodecahemidodecacron.
There are 2 more hemi Dual models, which between them represent 4 of the Semi-Regular Non-Convex Polyhedra, so we now have the whole 9 covered. I haven’t made either yet, but a drawing of the first one (Small Dodecahemicosacron / Great Dodecahemicosacron) is available on the internet:
And these are the two polyhedra of which it’s the dual:
The other one (Great Icosihemidodecacron / Great Dodecahemidodecacron) doesn’t seem to be available anywhere, which is not altogether surprising since it’s a bit of a monster to make: it’s basically the decagonal one above, but made with decagrams (stars) rather than plain decagons – which makes it much harder to fit together.
Just for the record, though, these are the polyhedra for which it is the dual:
That’s quite enough hard thinking (and tongue-twisting) for the moment. There’s a complete record of the job of building these shapes in the Details section, but now we’ll move on to Duals of the Prisms & Antiprisms.