If we go back to the early Polyhedra we notice there are curious and beautiful connections between them. Among the five Platonic solids we can show this by listing faces and vertices:
- Hexahedron (Cube): 6 faces, each with 4 edges; 8 vertices with 3 faces at each
- Octahedron: 6 vertices with 4 faces at each; 8 faces, each with 3 edges
All these 6s and 4s, 8s and 3s, seem to indicate a close relationship between these shapes: in fact they tell us that for every face of the cube there’s a vertex in the octahedron, and for every vertex of the cube there’s a face on the octahedron. This effect is called Duality and it means we can combine the two in a beautiful compound:
We can do the same with the Dodecahedron and Icosahedron, which are duals of each other. Here the numbers are 12 (faces and vertices) and 20 (vertices and faces). It’s worth stopping a moment just to visualise each of the underlying solids here: it helps you appreciate the beauty of the compound even more.
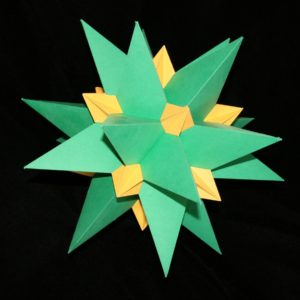
Even more impressive is to do this with the non-convex polyhedra. The Great Stellated Dodecahedron and Great Icosahedron are duals of each other, and here is the compound:
We could do the same with the Small Stellated Dodecahedron and the Great Dodecahedron, but if you do the maths right it turns out that one ends up completely inside the other…so we won’t bother with that one! (One of the standard textbooks on all this gives an alternative, but we won’t go there just yet.)
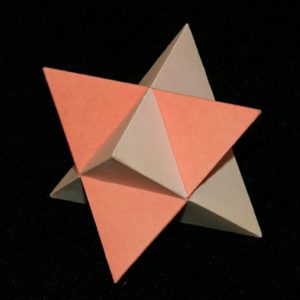
You may be asking – what about the Tetrahedron, the simplest one of them all? Does it have a dual? Well the Tetrahedron has 4 faces and 4 vertices, so it’s self-dual: the dual of a Tetrahedron is…a Tetrahedron! We can put two of them together in a rather pretty star-form known as a Stella Octangula – literally a star with 8 points:
It’s worth remembering the Stella Octangula: it pops up in all sorts of mediaeval drawings, it can be fitted perfectly into a cube, and it’s actually the first and only stellation of the Octahedron.
So having made a start down this strand, let’s now move on to More about Compounds.