I had an idea that not much work has been done stellating the 13 Semi-Regular (Archimedean) Solids, but I find on a careful reading of Wenninger’s Polyhedron Models that once again he got there long before me. But even he only really worked on the Cuboctahedron, and he was rather dismissive of the rest: and I did after all have my ‘Aha!’ moment all on my own. So I reckon I can take a little credit for what follows.
I actually looked at the Truncated Cube first, but we’ll kick off here with the Truncated Tetrahedron, on the grounds that (a) it’s the baby of the set, and (b) even then, it illustrates most of the issues that confront us with the more complex projects.
To begin with, we’ll recall what the Truncated Tetrahedron looks like:
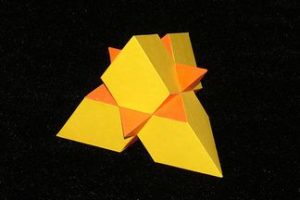
Looking at the basic shape some aspects of the stellation process are fairly obvious. Those orange hexagons will extend up and cover the yellow triangles, making little orange peaks which restore the shape of the original tetrahedron. Meanwhile the yellow triangles themselves will extend in some way above the orange faces. But what will happen across the orange edges? The result is quite hard to visualise until you do the geometry, but it ends up with the yellow now dominating while the orange becomes only a series of ‘nicks’ and ‘peaks’ round the sides:
I think this is an very beautiful shape, the more so if you manage to make it with really crisp edges and corners. There’s something delightful in being able to trace your finger round the nicks and peaks; and also a deeper feeling of awe as you consider those yellow parts. They appear to come in pairs, with each pair crossing the other like a couple of sticks of American butter (butter is sold in sticks in America, not blocks like everywhere else.)
As for what comes next: a further stellation will obviously fill in those little ‘nicks’, but there aren’t any other places where we can extend any of these these planes and have them meet: all the faces you’d think of extending turn out to run off to infinity. So we can’t extend the yellow blocks, or the little orange pyramids at all, and the only change here is to fill in the ‘nicks’. Happily, though, this clears out some of the clutter, and it leaves us with a pretty compound of a small tetrahedron locked into a bigger one – rather like an unbalanced Stella Octangula. Here is the Second Stellation of the Truncated Tetrahedron, rendered in green and lilac:
Are there any other Stellations of the Truncated Tetrahedron? Well no, and even the second one feels like a cheat because we didn’t extend all of the faces this time. But you can argue it differently and take the discussion in at least two different directions. If you drop a view that everything must be extended outwards at every step, and you also allow a more complex process of taking all the subsets of the three-dimensional ‘cells’ that the faces define, a lot more becomes possible.
The masters in this topic (Miller, Coxeter and Wenninger) take this more adventurous approach, and after some thought I’m sure they’re right. But it took me a while to deconstruct and rebuild my thinking – so to lay out exactly what the arguments are, here’s the list of my five stellations of the Truncated Tetrahedron:
| Stellation | What it consists of | The logical step that gets you there |
| 1 | The yellow and orange parts shown above | Obtained by extension: extending across every edge in the stellation diagrams to the next part of the diagram |
| 2 | The green and purple parts shown above | Obtained by extension, but only where this is possible (i.e. filling in the ‘nicks’.) If we can’t extend (i.e. on the orange faces or the big sides of the yellow ones, just leave them as they are. |
| 3 | Simply the necklace of small orange/purple tetrahedra, with vacant space between | Obtained as a sub-set of the three-dimensional cells |
| 4 | Simply the yellow cells, with a hole between them | Again, obtained as a sub-set of the three-dimensional cells |
| 5 | ‘Stellation to Infinity’ | A rather different approach that extends the parallel faces and edges of the yellow and orange cells |
I haven’t made the last three of these yet, and the idea of ‘Stellation to Infinity’ is quite a stretch… but don’t worry. Let’s hurry on and look at a rather more advanced set: the Stellations of the Truncated Octahedron.